Maths - Essay Example

The equation of a line or curve expressed in polar coordinates is known as a polar equation, and is usually written with r as a function of θ. A polar line/curve is symmetric about the 0°/180° ray if replacing θ by −θ in its equation produces in an equivalent equation, symmetric about the 90°/270° ray if replacing θ by π−θ produces an equivalent equation, and symmetric about the pole if replacing r by −r produces an equivalent equation. Any polar line/curve can be rotated α° counterclockwise about the pole by substituting θ−α in the equation for θ.

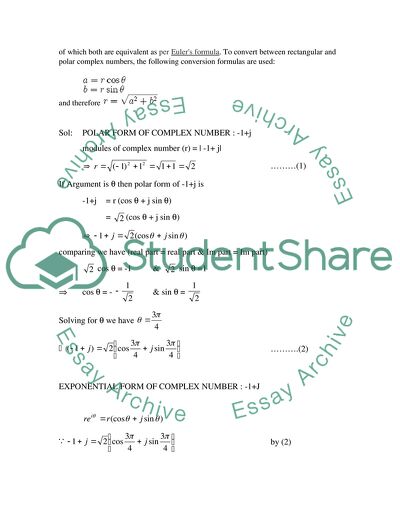
In mathematics, the complex plane is a way of visualising the space of the complex numbers. It can be thought of as a modified cartesian plane, with the real part represented in the x-axis and the imaginary part represented in the y-axis. The x-axis is also called the real axis and the y-axis is called the imaginary axis. The complex plane is sometimes called the Argand plane for its use in Argand diagrams. Its creation is generally credited to Jean-Robert Argand, although it was first described by Norwegian-Danish land surveyor and mathematician Caspar Wessel.

The concept of the complex plane allows a geometric interpretation of complex numbers. Under addition, they add like vectors, and the multiplication of complex numbers can be expressed simply using polar coordinates, where the magnitude of the product is the product of those of the terms, and the angle from the real axis of the product is the sum of those of the terms. Laplace transform is a powerful technique for analyzing linear time-invariant systems such as electrical circuits, harmonic oscillators, optical devices, and mechanical systems, to name just a few.

Given a simple mathematical or functional description of an input or output to a system, the Laplace transform provides an alternative functional description that often simplifies the process of analyzing the behavior of the system, or in