Quantitative Methods for Portfolio Analysis - Assignment Example

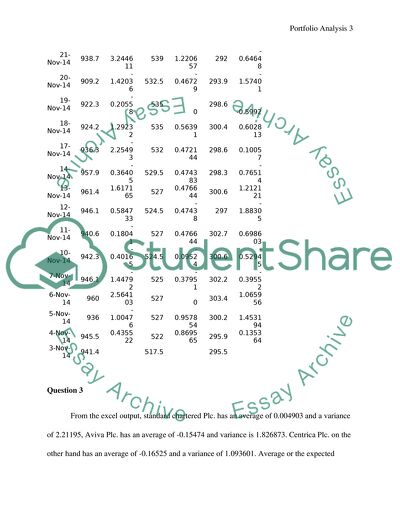
Portfolio Analysis Introduction Portfolio analysis is a technique that shows the investor how to combine different assets so as to maximize the returns generated by the assets as well as risk diversification (Huang 2010, p. 139). Lee (2002, p. 234) states that a portfolio on the other hand is the different combination of assets that are held by the investor for investment purposes, an investor can also decide to hold single assets rather than a portfolio also known as stand -alone assets. This report will seek to perform an analysis on the stock prices and the expected returns for the three companies based in the United Kingdom. The three companies that will form the basis of our analysis are Standard Chartered Plc., Aviva Plc. and Centrica plc. The companies operate in different industries and they also command different market prices. The time frame for our analysis will be from November 3, 2014 to December 2, 2014 Question 2 (Data for the Study) Standard Chartered Aviva Plc. Centrica Plc. Date Price RETURNS Price RETURNS Price RETURNS 2-Dec-14 940.3 2.552078 500 0.120144 285.1 1.242898 1-Dec-14 916.9 -2.15559 499.4 -1.69291 281.6 -1.05411 28-Nov-14 937.1 -0.31911 508 0 284.6 -1.07751 27-Nov-14 940.1 0.405853 508 0.197239 287.7 0.524109 26-Nov-14 936.3 -0.06404 507 -0.19685 286.2 0.52687 25-Nov-14 936.9 0.289017 508 -0.39216 284.7 -0.38488 24-Nov-14 934.2 -0.47939 510 -5.38033 285.8 -2.12329 21-Nov-14 938.7 3.244611 539 1.220657 292 -0.64648 20-Nov-14 909.2 -1.42036 532.5 -0.46729 293.9 -1.57401 19-Nov-14 922.3 -0.20558 535 0 298.6 -0.5992 18-Nov-14 924.2 -1.29232 535 0.56391 300.4 0.602813 17-Nov-14 936.3 -2.25493 532 0.472144 298.6 0.10057 14-Nov-14 957.9 -0.36405 529.5 0.474383 298.3 -0.76514 13-Nov-14 961.4 1.617165 527 0.476644 300.6 1.212121 12-Nov-14 946.1 0.584733 524.5 -0.47438 297 -1.88305 11-Nov-14 940.6 -0.18041 527 0.476644 302.7 0.698603 10-Nov-14 942.3 -0.40165 524.5 -0.09524 300.6 -0.52945 7-Nov-14 946.1 -1.44792 525 -0.37951 302.2 -0.39552 6-Nov-14 960 2.564103 527 0 303.4 1.065956 5-Nov-14 936 -1.00476 527 0.957854 300.2 1.453194 4-Nov-14 945.5 0.435522 522 0.869565 295.9 0.135364 3-Nov-14 941.4 517.5 295.5 Question 3 From the excel output, standard chartered Plc. has an average of 0.004903 and a variance of 2.21195, Aviva Plc. has an average of -0.15474 and variance is 1.826873. Centrica Plc. on the other hand has an average of -0.16525 and a variance of 1.093601. Average or the expected returns of the three assets computed separately shows the amount that the investor is likely to obtain if he decides to invest in either of the three companies (Markowitz, & Todd 2000, p. 124). Variance also known as the absolute risk is a measure of the risk associated with a given asset. Elton (2010) postulates that assets with the lowest risk are preferable as compared to the others depending on the risk preference of the investor. Centrica Plc. Has the lowest risk at the given level of returns, in regards to the returns Standard Chartered has the highest returns at a given level of risk. Since different investors have different preferences in terms of the risk and returns of an asset, an investor may prefer the asset with the highest risk and high returns or low risk and low returns (Johnson 2014, p. 109). Question 4 A variance-covariance matrix is a matrix that contains the variance and covariance related with many stocks. The diagonal elements of the matrix contains the variances of the stocks whereas the other elements that are not in the diagonal represents the covariance between the different stocks. The variance-covariance matrix for the three stocks is given as follows; VARIANCE-COVARIANCE MATRICK Stan chart Aviva Centrica Stan chart 2.21195 0.48 0.463759 Aviva 0.48 1.826873 1.826873 Centrica 0.463759 1.826873 1.093601 Correlation standard chartered-Aviva 0.238781 Correlation standard chartered-Centrica 0.298178 Correlation Aviva-Centrica 0.582136 Correlation refers to the relationship between different stocks. A negative correlation shows that the two stocks prices move in different direction whereas the positive correlation as indicated from the excel output of the correlations between the stocks implies that the stock prices move in the same direction. Question 5a. Combinations Weights-Aviva Weights-Centrica Return standard deviation 1 0 1 -0.154737484 1.045753851 2 0.1 0.9 -0.325999471 1.025765236 3 0.2 0.8 -0.497261457 1.017980339 4 0.3 0.7 -0.668523444 1.022677893 5 0.4 0.6 -0.83978543 1.039688715 6 0.5 0.5 -1.011047416 1.068424833 7 0.6 0.4 -1.182309403 1.107974311 8 0.7 0.3 -1.353571389 1.157229006 9 1 0 -1.557882381 1.351618794 5b. Combinations Weight- standard chartered Weight-Aviva Returns Standard deviation 1 0 1 -0.154737484 1.351618794 2 0.9 0.1 -0.011060904 1.377079508 3 0.8 0.2 -0.027024968 1.281531403 4 0.7 0.3 -0.042989033 1.204107122 5 0.6 0.4 -0.058953097 1.148477968 6 0.5 0.5 -0.074917162 1.117902404 7 0.4 0.6 -0.090881226 1.114444428 8 0.3 0.7 -0.106845291 1.138351194 9 1 0 0.00490316 1.487262473 Question 7a. An efficient portfolio refers to the portfolio that gives the highest expected return at any level of risk or the lowest degree of risk for any expected return, an investor tends to hold the assets that minimizes risk. Markowitz efficient frontier is the efficient set of investment and any asset that lies on any point on the frontier dominates all the other points on the feasible set. Therefore the assets lying on the frontier are preferable due to the fact that they have high returns at a given degree of risk or low risk at a given level of expected returns while those lying outside the frontier are regarded as inefficient assets and they are considered unattractive to the investors (Kariya 2013, p. 78). Question 7b. Based on the results obtained from the Markowitz efficient frontier, investing in a portfolio is preferable than an individual stock. Investing in a portfolio has the advantage of risk reduction as compared to an individual stock. Risk reduction arises as a result of combining the assets whose expected returns are not perfectly correlated to reduce the aggregate risk of the portfolio (Fong 1980, 149). Based on the three companies, the most preferable correlation is Standard Chartered Plc. and Aviva Plc. The two assets have a less correlation coefficient and therefore holding them in a portfolio greatly reduces their risk. Question 8a A risk free asset is an asset whose actual returns are equal to the expected returns, this implies that the asset shoes no variability in the expected returns and as a result, the variance and standard deviation is zero (Markowitz, & Todd 2000, p. 129). Although the normal returns are constant, the actual returns may be uncertain due to inflation and other unforeseen occurrences. Combining a risk free asset with a risky assets gives a covariance and correlation value zero. Lending and borrowing at a risk free arte and the MEF is explained by the separability theorem which states that financing and investment decisions bare separate and distinct and this implies that the investors can invest in the same securities regardless of how they obtained the capital (Johnson 2014, p. 201). The investors will imitate the market portfolio by purchasing the assets that constitute the market portfolio and the risk free asset. Investing in the market portfolio and the risk free rate is joined by the capital market line. Question 8b. The decision to hold a portfolio as opposed to an individual asset will not change due to the existence of a risk free rate instead the feasible set will expand therefore changing the location of the efficient frontier. The assets in the portfolio will also increase thus, the optimal solution will be at the point of tangency between the efficient frontier and the capital market line. Existence of a risk free asset has an advantage in the sense that the investment will have a zero risk and will cut the Y-axis at the rate of return equivalent to the risk free rate. Reference List HUANG, X. (2010). Portfolio analysis: from probabilistic to credibilistic and uncertain approaches. Berlin, Springer Verlag. LEE, C. F. (2002). Advances in investment analysis and portfolio management. Volume 9 Volume 9. Amsterdam, JAI. http://search.ebscohost.com/login.aspx?direct=true&scope=site&db=nlebk&db=nlabk&AN=199088. MARKOWITZ, H., & TODD, G. P. (2000). Mean-variance analysis in portfolio choice and capital markets. New Hope, Pa, F.J. Fabozzi Associates. ELTON, E. J. (2010). Modern portfolio theory and investment analysis. Hoboken, NJ, J. Wiley & Sons. JOHNSON, R. S. (2014). Equity markets and portfolio analysis. Hoboken, New Jersey, Bloomberg Press. http://search.ebscohost.com/login.aspx?direct=true&scope=site&db=nlebk&db=nlabk&AN=801707. KARIYA, T. (2013). Quantitative methods for portfolio analysis: mtv model approach. [S.l.], Springer. FONG, H. G. (1980). Bond portfolio analysis. Charlottesville, VA, Financial Analysts Research Foundation. Read More